FPL analysis: attacking combinations from the same club

Over the last week following the relaunch of the new Fantasy Premier League (FPL) season, the dedicated participants of the game have begun sharing drafts of teams that they may enter for Gameweek 1 on Twitter. A factor of this early planning that has struck me has been the willingness of some managers to ‘double-up’ on attacking assets from the same team; for example, selecting Aguero and De Bruyne from Manchester City is a popular move that I have seen.

At an instinctive level, this strategy feels foreign to me in the early stages. There is a wealth of attacking talent in the Premier League, and limited spaces in an attacking line-up. Choosing two assets from a particular club leaves at most five midfield / forward positions open for other clubs. Considering the budget restrictions, proven attacking threats from some elite clubs will inevitably have to be left out. Intuitively, I tend to hedge my bets between teams rather single-club players historically.

The doubling-up strategy is by no means universally accepted. I conducted a straw poll on Twitter to see how many managers favoured using dual attacking assets from the same team.

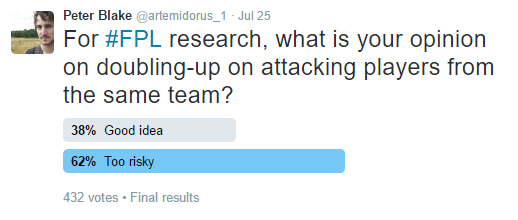
Although nearly two-thirds agreed with my instincts that it was too risky, most of the comments I received were from those who fell into the ‘it depends’ camp or were advocates espousing past successes such as Bale/van der Vaart, Vardy/Mahrez and Rooney/van Persie. One particular comment on Fantasy Football First was particularly interesting:

“If City create 7 chances to score, while Arsenal only 5, then having a player from each team only gives you an average of 6 potential chances per player, while having two players from City gives you 7 potential chances per player.”

Whilst this is of course true, it works both ways: having a double Arsenal attack under such circumstances would only give you five chances, two less than the City players.

Ultimately, the decision comes down to a personal judgement on a game by game basis, and I already knew before embarking on the following analysis that there would be no ‘silver bullet’ strategy which could be recommended. If you’re looking for a recommendation to emerge from this post, I’d stop reading now. FPL is a game of probabilities, and the best we can do is to try to use our best judgement to make a favourable call. Nonetheless, I was curious to see what the recent history indicated, to see what the likelihood of two players from the same team producing returns were.

Methodology

For this analysis I have restricted the sample to midfield and attacking players only. The analysis looks at 380 games from each of the 2014/15 and 2015/16 seasons, totalling 1,520 team performances from 760 games. I have determined six or points to be the benchmark for a successful game from an FPL perspective.

A frequent occurrence

Figure 1: Occurrences of midfielders or forwards from the same team scoring six or more points in the same game (n=1,520)

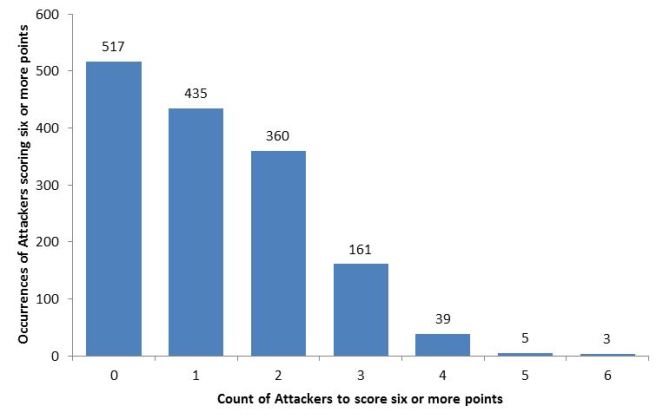
There have been 568 occasions where two or more attackers from the same team have scored six or more points in the last two seasons, which equates to 37.4% of available opportunities. Put another way, on an average gameweek, 3.7 of the 10 teams playing will have multiple scorers of a good point totals. It is more frequent than a team having no players above six points (34.0%) or a team having only one such player (28.6%) although obviously less than these combined.

Looking at the leading combinations over the last couple of seasons will no doubt bring back fond memories for the FPL managers reading.

Figure 2: Leading combinations, 2014/15 (both players must have scored at least six points)

Figure 3: Leading combinations, 2015/16 (both players must have scored at least six points)

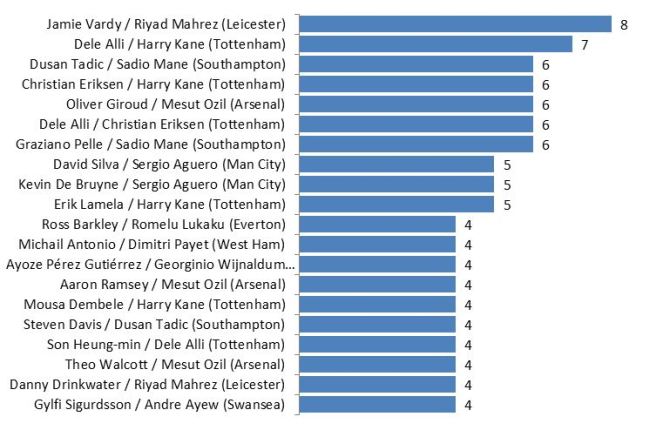
What is notable about this data is how frequently some combinations deliver. Fabregas / Hazard (2014/15) and Vardy / Mahrez (2015/16) scored at least six points each in 21% of the title-winners’ games. Another interesting factor is how some clubs get into a rhythm where it would even be beneficial to have three players from the attacking line-up in your team: three variations of Kane / Eriksen / Alli for Tottenham in 2015/16 and Fabregas / Hazard / Costa for Chelsea in 2014/15 all feature in the top seven and four combinations in their respective seasons.

Whilst the data is compelling and suggests that two or more players are quite likely to score a good amount of points, it is interesting to delve a little deeper into the data to discover what this really means. For a start, and crucially, we don’t know which two attackers from a team are going to score the points. Taking the earlier anecdote about Manchester City, whilst managers are doubling up on Aguero and De Bruyne the assumption is that it will be these two players who score the points and not any of Toure, Navas, Sterling, Nolito, Silva, Fernandinho, Fernando, Delph, Nasri, Gundogan, Zinchenko, Bony or Iheanacho.

Another factor to consider is that the ‘two-players-scoring-six-or-more’ probability increases the more the leading point scorer accumulates.

Figure 4: Count of a team’s attacking players to score six or more points, by points of the leading scorer (n=1,520)

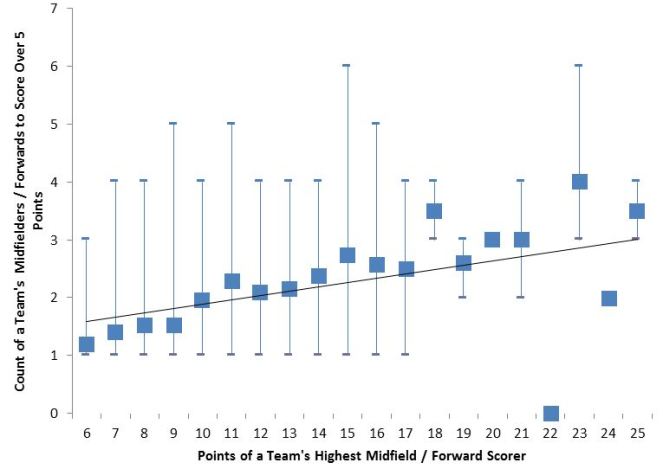
The chart above shows the maximum and minimum number of attackers who have scored six or more points (represented by the top and bottom of the vertical lines) in relation to how many points the leading point scorer has scored. For example, when the leading point scorer for a team has scored ten points, his team provide between one (himself) and four players who have scored six or more points. The blue squares represent the average number of players, or what we can realistically expect.

Looking at this data we see that, returning to the Manchester City example, if Aguero scores six points and is a leading scorer for the team, City will have an average 1.19 attackers scoring six points. Considering the 1 in the 1.19 is Aguero himself, this means that 0.19 of his colleagues will score six points, or put another way there is about a one in five chance of one further attacker scoring six. Moving further up the scale, if Aguero goes on the rampage and returns a 19 point haul, then we can expect a further 1.6 of his teammates to also score six or more.

If we’re looking for a threshold of when two attackers from the same team are likely to score six or more points, then it is when the leading point scorer contributes ten points. At this stage, his team will have an average of 1.97 players scoring six or more, which practically means the leading scorer himself plus 0.97 others, or one other in nearly all cases. Therefore, the lesson from this data is that, before we even get to guessing which of the two attackers to select, we must be confident that one of them is going to score at least ten points if they are both likely to score the minimum of six points.

Falling Efficiency

So let’s assume that we defied the odds, which to date are:

• Picking one of the 3.7 teams each week which will have two high point scorers
• The two attackers you have selected are the correct two, which in the Manchester City case of 15 attacking players amounts to a one in 105 chance (all things being equal and making no assumptions about who is more likely to score, which of course is not reflective of the fact that we know intuitively and from past form that Aguero is probably the most likely to score, but we won’t add that variable at this point).

Assuming that our earlier example from the introduction has proved fruitful and De Bruyne and Aguero have both come home with six or more points each. One further thing we might want to consider is whether it is efficient to select them both. Looking at the points scored per million of each team’s leading point scorer vs. the combined points per million of the leading two players, we see that in all cases where the leading attacker has scored over two points, the points per million total is lower for both players than it is for the leading attacker.

Figure 5: Points per million of leading point scorer vs. points per million of top two leading point scorers combined (n=1,520)

An illustrative example of this would be if, as previously mentioned, Aguero scores ten points and De Bruyne scores six. At £13.0m, Aguero will contribute 0.77 points per million (=10/13). If we add De Bruyne (£10.5m) and his six points to the equation, we see that the points per million drops to 0.68 (=10+6/13+10.5). This is what we have seen replicated across the game in the last two seasons (see figure 3); that the efficiency of two attackers from the same club – the leading points scorers that week – is lower than for a single player.

It should be noted that in the above example, in cases where points were tied for 1^st and 2^nd or 2^nd and 3^rd, the more expensive player was afforded the position of leader, which could affect the points per million calculations in the instances where it happened. Regardless, the data raises an interesting theoretical argument: why pick a second attacker from the same club when you could pick a probable leading attacker from another club? Put another way, why spend £10.5m on De Bruyne to compliment Aguero when you could spend similar money on Sanchez, Hazard, Martial, etc?

Figure 6: Points of the leading attacker vs. Points of the 2^nd highest scoring attacker (n=1,520)

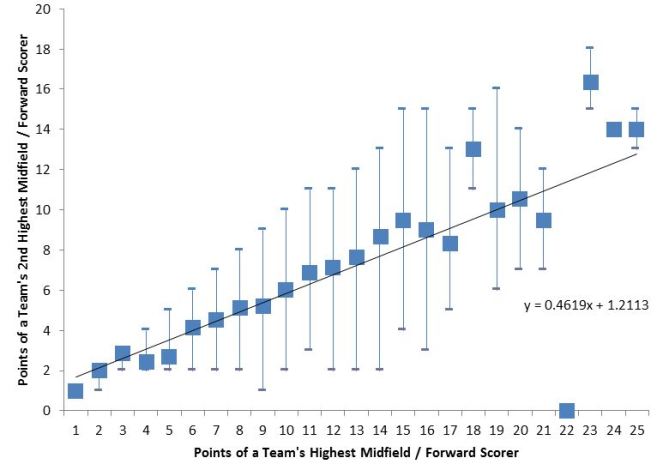
The chart above shows the average points scored by the second leading attacker relative to the leading attacker (as well as the highs and lows of the historical data). The equation on the chart is the formula for the trendline, which suggests that the second attacker will likely score less than half of the leading attacker’s points (0.46) plus 1.2. Therefore, in our example, if Aguero scores ten, then De Bruyne should score just under half (4.6) plus 1.2, which rounded up equals six. In this hypothetical, would it not be more favourable to go after leading points scorers from different teams?

Summary

The reason of course is that this argument assumes that the leading points scorers of each team will be roughly equal. However, it just as likely that Sanchez will score equal to Aguero and dwarf the points of De Bruyne, as it is that De Bruyne’s six points will beat Sanchez, who may only score five. At which point we’re back to the start, as we expected to be: there is no ‘silver bullet’ strategy evident from the data because ultimately the circumstances of each game are unique. The only real course is to trust your judgement; if you think that City have a realistic chance of scoring multiple times and Arsenal are likely to be shut out, then it is very reasonable to pick a second City attacker over the leading Arsenal attacker.

What we have discovered however is that dual-attacker options from single clubs does pay dividend 37% of the time, however this is generally conditional on the leading player securing a double-point haul and always conditional on the FPL manager being able to divine which two assets are most likely to bring home the points, which is unquestionably the most difficult factor to master. Additionally, even in the best case scenarios, the efficiency of your team will likely decrease as a result. In summary, considering these stats it initially seems like success from this strategy is unlikely, that is until we consider that picking the right players from a variety of teams is equally as unlikely. Therein lays the brilliance and addition of FPL: every additional point you earn is, in many ways, a defiance of the odds that should be celebrated.